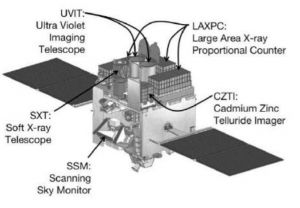
2016年IOAA理论第12题-Astrosat(Discarded)
本题目目前没有解答。要不要你来试试! |
|
英文题目
(T12) AstroSat
India astronomy satellite, AstroSat, launched in September 2015, has five different instruments.
In this question, we will discuss three of these instruments (SXT, LAXPC, CZTI), which point in the same direction and observe in X-ray wavelengths. The details of these instruments are given in the table below.
| Instrument | Band [keV] | Collecting Area [m2 ] | Effective Photon Detection Efficiency | Saturation level [counts] | No. of Pixels |
|---|---|---|---|---|---|
| SXT | 0.3-80 | 0.067 | 60% | 1500(total) | 512x512 |
| LAXPC | 3-80 | 1.5 | 40% | 50000 (in any one counter) or 200000 (total) | --- |
| CZTI | 10-150 | 0.09 | 50% | --- | 4 x 4096 |
You should note that LAXPC energy range is divided into 8 different energy band counters of equal bandwidth with no overlap.
(T12.1) Some X-ray sources like Cas A have a prominent emission line at 0.01825 nm corresponding to a radioactive transition of 44Ti . Suppose there exists a source which emits only one bright emission line corresponding to this transition. What should be the minimum relative velocity (𝑣) of the source, which will make the observed peak of this line to get registered in a different energy band counter of LAXPC as compared to a source at rest?
These instruments were used to observe an X-ray source (assumed to be a point source), whose energy spectrum followed the power law,
𝐹(𝐸) = 𝐾𝐸−2⁄3 [in units of counts/keV/m2/s ]
where 𝐸 is the energy in keV, 𝐾 is a constant and 𝐹(𝐸) is photon flux density at that energy. Photon flux density, by definition, is given for per unit collecting area (m2 ) per unit bandwidth (keV) and per unit time (seconds). From prior observations, we know that the source has a flux density of 10 counts/keV/m2 /s at 1 keV, when measured by a detector with 100% photon detection efficiency. The “counts” here mean the number of photons reported by the detector. As the source flux follows the power law given above, we know that for a given energy range from 𝐸1 (lower energy) to 𝐸2 (higher energy) the total photon flux (𝐹T) will be given by
𝐹T = 3𝐾 (𝐸21⁄3 − 𝐸11⁄3) [in units of counts/m2/s ]
(T12.2) Estimate the incident flux density from the source at 1 keV, 5 keV, 40 keV and 100 keV. Also estimate what will be the total count per unit bandwidth recorded by each of the instruments at these energies for an exposure time of 200 seconds.
(T12.3) For this source, calculate the maximum exposure time (𝑡S ), without suffering from saturation, for the CCD of SXT.
(T12.4) If the source became 3500 times brighter, calculate the expected counts per second in LAXPC counter 1, counter 8 as well as total counts across the entire energy range. If we observe for longer period, will the counter saturate due to any individual counter or due to the total count? Tick the appropriate box in the Summary Answersheet.
(T12.5) Assume that the counts reported by CZTI due to random fluctuations in electronics are about 0.00014 counts per pixel per keV per second at all energy levels. Any source is considered as “detected” when the SNR (signal to noise ratio) is at least 3. What is minimum exposure time, 𝑡𝑐 , needed for the source above to be detected in CZTI? Note that the “noise” in a detector is equal to the square root of the counts due to random fluctuations.
(T12.6) Let us consider the situation where the source shows variability in number flux, so that the factor 𝐾 increases by 20%. AstroSat observed this source for 1 second before the change and 1 second after this change in brightness. Calculate the counts measured by SXT, LAXPC and CZTI in both the observations. Which instrument is best suited to detect this change? Tick the appropriate box in the Summary Answersheet.